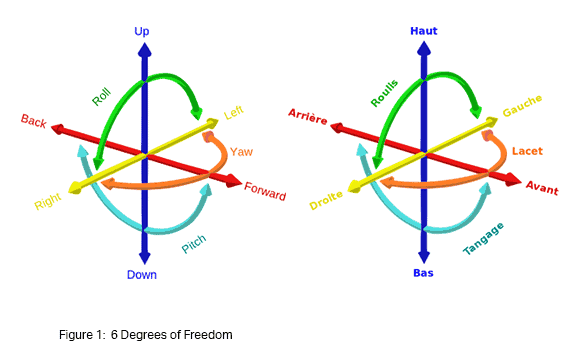
Serapid employs several technologies to create stable lifting platforms. These can include Scissor, Wallet, and Lambda mechanisms and simple bearing guides along with the inherent stability of the Rigid Chain product. For a platform, it must not move in any of three directions and three rotations. In engineering terms, we are removing degrees of Freedom (Figure 1).
A single SERAPID lifting column (such as the Chainmast or LinkLift) eliminates one degree of freedom. The chain controls the up/down position of the platform on top of the chain. A platform sitting on top of a single chain column is still free to move laterally (Left/Right and forward/backward) as well as to rotate or twist (Pitch, Roll, Yaw). (Figure 2)
Adding a second lifting column eliminates a second, rotational, degree of freedom. If the two columns are left and right of each other then roll is eliminated. It can still spin or yaw, and pitch, as well as move laterally. A third or fourth column is added out of line with the first two, make the platform stable. Much like a stool or a chair, it does not roll or pitch. Still, the platform is not stable. It can still twist or yaw. It can also sway in the Right/Left and Forward/Backward directions. At this point, external stabilization is required. There are still 3 degrees of freedom to constrain. External guidance of the platform is required to constrain these three.
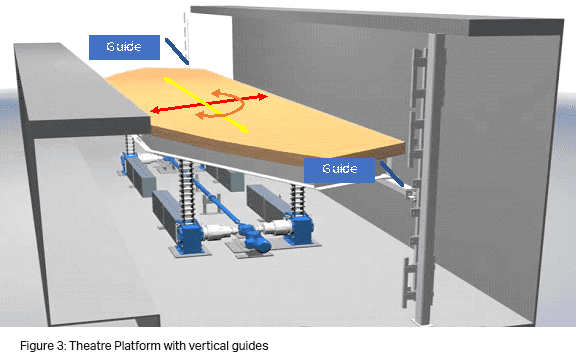
A simple method is to place two vertical guides (elevator guides) on the platform. At the guide points, they prevent any motion in the lateral directions (Left/Right or Forward/Backward) at the guides. The combination of the two also prevents twist or yaw in the platform (Figure 3). In some cases, it is not possible to have external guides for the platform. Then other methods are required
 F
F
A scissor mechanism provides stability by constraining these three degrees of freedom. A single scissor is simply 2 bars crossed in an X shape. The center of the X has a pivot. On one side of the X, the top and bottom extents have pivots. The other side of the X has both pivots and sliders (Figure 4). When moving, the upper pivots always remain vertically above the lower pivots. The height between the upper and lower pivots is the same for both sides of the scissor.
Because the pivot pairs are always vertical, they constrain one lateral degree of freedom. Because the height of the two pairs is always the same, it eliminates a rotational degree of freedom. A single scissor could sway so a second one is added with a lateral stabilizer. This eliminates a third and lateral degree of freedom. The two scissors are tied with the lateral stabilizer and cannot be at different heights. This eliminates a fourth and rotational degree of freedom. The pair of scissors also eliminates the fifth and rotational degree of freedom (twist or yaw). The complete scissor mechanism constrains 5 degrees of freedom and the only one remaining is constrained by the lifting mechanism. In this example, the Serapid Rigid Chain performs this function. (Figure 4)
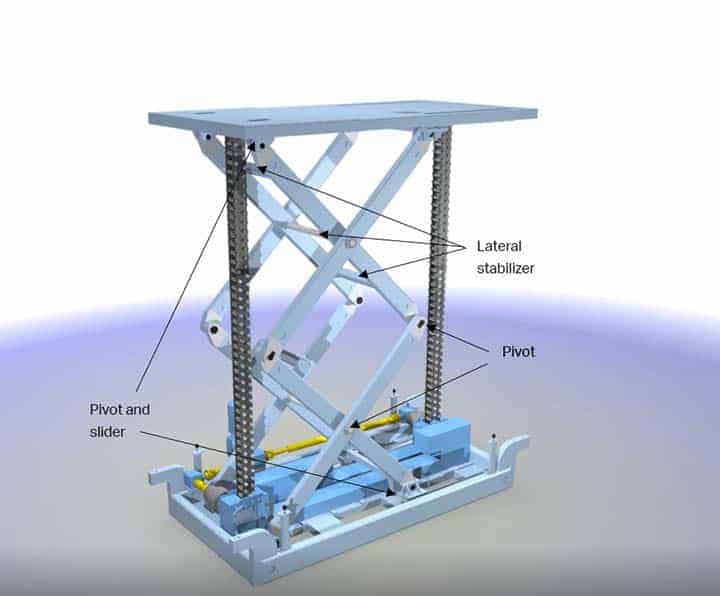
Figure 4: Scissors eliminates 5 degrees of freedom.
A full scissor mechanism is relatively large and consumes valuable space beneath the platform. Functions of the scissor can be broken out into multiple and smaller mechanisms. A wallet and lambda (Figure 5 and Figure 6) are derivative of the scissor.

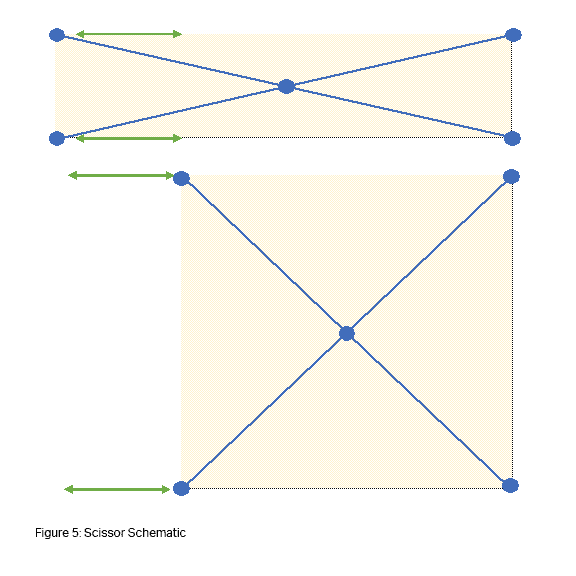
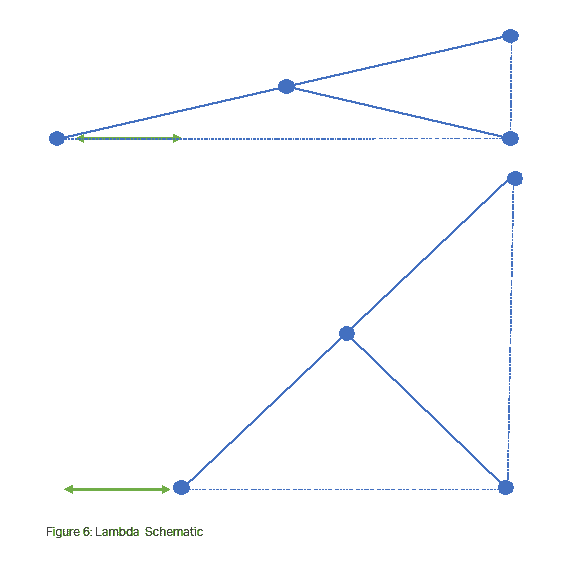
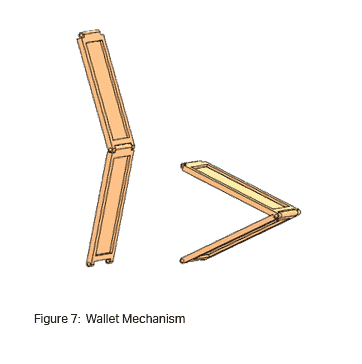
The lambda causes an upper pivot point to remain always vertical above the corresponding lower pivot. It constrains one lateral degree of freedom. The wallet mechanism constrains one lateral and two rotational degrees of freedom. Two wallets and a lambda can be used together to constrain 5 degrees of freedom, like the scissor mechanism. Though more complex, it has the benefit of allowing more design freedom in placing the components of the lifting mechanism (Figure 7).

by Robert C. Adams, PhD, PE
Director of R&D and Engineering, SERAPID